Unit 6 Radical Functions Answer Key

Embark on an enlightening journey into the realm of radical functions, where we unveil the mysteries of these intriguing mathematical expressions. Delving into the depths of unit 6 radical functions answer key, this comprehensive guide illuminates the intricacies of radical functions, providing a profound understanding of their nature, applications, and significance in various fields.

Through a series of meticulously crafted explanations, examples, and practice problems, this answer key empowers learners to conquer the challenges of radical functions. By mastering the concepts Artikeld within, students will gain the confidence to tackle complex radical expressions, graph radical functions with precision, and harness their power in real-world applications.

Definition and Overview

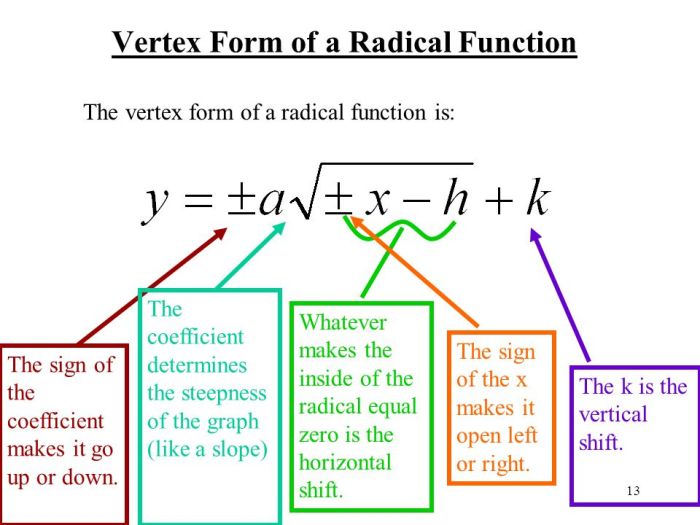
Radical functions are a type of mathematical function that involve the square root of a variable.

Examples of radical functions include:

$f(x) = \sqrtx$
$g(x) = \sqrtx^2 + 1$
$h(x) = \sqrt[3]x + 2$

The domain of a radical function is the set of all real numbers for which the expression inside the radical is non-negative. The range of a radical function is the set of all real numbers that are greater than or equal to zero.

Examples of Radical Functions

Some common examples of radical functions include:

$f(x) = \sqrtx$
$g(x) = \sqrtx^2 + 1$
$h(x) = \sqrt[3]x$

These functions are all defined for all real numbers, and they all have a range of $[0, \infty)$.

Simplifying Radical Expressions

Simplifying radical expressions involves transforming them into their simplest form, making them easier to understand and work with.Simplifying radical expressions requires understanding radical properties, such as:

Multiplying radicals with the same index

“`√(a)

√(b) = √(ab)

“`

Dividing radicals with the same index

“`√(a) / √(b) = √(a/b)“`

Raising a radical to a power

“`(√(a))^n = √(a^n)“`Methods for simplifying radical expressions include:

Removing perfect squares from under the radical

“`√(4) = √(2^2) = 2“`

Rationalizing the denominator of a radical

“`√(2/3) = (√(2/3))

(√(3/3)) = √(2/9) = 2/3

“`

Combining like radicals

“`√(2) + √(2) = 2√(2)“`Simplifying radical expressions helps in solving equations, simplifying expressions, and understanding mathematical concepts.

Graphing Radical Functions

Graphing radical functions involves understanding their unique characteristics and following specific steps to accurately represent them on a coordinate plane. These functions exhibit distinct behaviors due to the presence of a radical expression within their equations.

Steps for Graphing Radical Functions

Determine the Domain:Identify the values of the independent variable for which the radical expression is defined. Exclude any values that make the radicand negative or result in an even root of a negative number.
Find Intercepts:Calculate the x-intercept by setting yto zero and solving for x. Similarly, find the y-intercept by setting xto zero and solving for y.
Plot Key Points:Choose additional points within the domain to evaluate the function and plot them on the coordinate plane. These points will help establish the shape of the graph.
Draw the Graph:Connect the plotted points smoothly to form the graph of the radical function. The graph may exhibit specific characteristics, such as symmetry or asymptotes, depending on the function.

Key Features of Radical Functions

Domain Restrictions:The domain of a radical function is limited to values that make the radicand non-negative.
Vertical Asymptotes:If the radical expression contains an even root, the graph will have a vertical asymptote at x= 0.
Horizontal Asymptotes:If the index of the radical is odd, the graph may approach a horizontal asymptote as xapproaches infinity.
Symmetry:Some radical functions may exhibit symmetry about the y-axis or the origin, depending on the nature of the radical expression.

Examples of Graphing Radical Functions

Consider the following radical function:

y= √( x+ 2)

Domain: x≥ -2 (radicand must be non-negative)

Intercepts: x-intercept: (-2, 0); y-intercept: (0, √2)

Key Points:(1, √3), (4, √6)

Graph:The graph of this function is a half-parabola opening upwards, with a vertical asymptote at x= -2.

Applications of Radical Functions

Radical functions have numerous real-world applications across various fields, including mathematics, physics, engineering, economics, and biology.

In mathematics, radical functions are used to solve equations, simplify expressions, and perform calculations involving roots and powers. They play a crucial role in trigonometry, calculus, and algebra.

Physics

Projectile motion: The trajectory of a projectile, such as a ball or rocket, can be described by a radical function that models the relationship between the height of the projectile and the time elapsed.
Electromagnetism: The magnetic force between two magnets is inversely proportional to the square root of the distance between them, making it a radical function.

Engineering, Unit 6 radical functions answer key

Bridge design: The strength of a bridge beam is proportional to the square root of its cross-sectional area, making it a radical function.
Fluid dynamics: The flow rate of a fluid through a pipe is proportional to the square root of the pressure difference, making it a radical function.

Economics

Consumer demand: The demand for a product is often related to its price by a radical function, with demand decreasing as price increases.
Market equilibrium: The equilibrium price of a product is the point where supply and demand intersect, which can be modeled by a radical function.

Biology

Population growth: The growth rate of a population is often modeled by a radical function, with growth slowing down as the population size increases.
Enzyme kinetics: The rate of an enzymatic reaction is proportional to the square root of the substrate concentration, making it a radical function.

These are just a few examples of the many applications of radical functions. Their ability to model various phenomena makes them essential tools in a wide range of disciplines.

Inverse Radical Functions

Inverse radical functions are functions that undo the operation of a radical function. In other words, if f(x) is a radical function, then its inverse function f^(-1)(x) will produce the input x when given the output f(x).

To find the inverse of a radical function, we can follow these steps:

Replace y with f(x) in the original function.
Solve the resulting equation for x.
Replace x with f^(-1)(y) in the solved equation.

Here are some examples of inverse radical functions:

The inverse of the function f(x) = sqrt(x) is f^(-1)(x) = x^2, since sqrt(x^2) = x.
The inverse of the function f(x) = cube root(x) is f^(-1)(x) = x^3, since cube root(x^3) = x.
The inverse of the function f(x) = 4th root(x) is f^(-1)(x) = x^4, since 4th root(x^4) = x.

Rationalizing Denominators

Rationalizing denominators involves rewriting a fraction so that the denominator is a rational number, that is, an integer or a fraction. This process is often used to simplify expressions or to prepare them for further calculations.There are two main methods for rationalizing denominators:

Conjugate Pairs

Multiply and divide the fraction by the conjugate of the denominator.
The conjugate of a binomial expression $a + b$ is \(a
b\).
For example, to rationalize the denominator of $\frac1\sqrt2 + 1$, we multiply and divide by \(\sqrt2

1\)

$$\frac1\sqrt2 + 1 \cdot \frac\sqrt2

1\sqrt2
1 = \frac\sqrt2
1(\sqrt2 + 1)(\sqrt2
1) = \frac\sqrt2
12
1 = \sqrt2
1$$

Squaring the Denominator

If the denominator is a binomial expression with a square root, square the denominator.
For example, to rationalize the denominator of \(\frac1\sqrt3

2\), we square the denominator

$$\frac1\sqrt3

2 = \frac1\sqrt3
2 \cdot \frac\sqrt3 + 2\sqrt3 + 2 = \frac\sqrt3 + 2(\sqrt3
2)(\sqrt3 + 2) = \frac\sqrt3 + 23
4 =
(\sqrt3 + 2)$$

Rationalizing denominators is a useful technique for simplifying expressions and making them more manageable for further calculations.

Complex Radical Expressions

Complex radical expressions are radical expressions that contain other radicals within their radicands. They can be simplified using the rules of radicals and the properties of complex numbers.

Operations on complex radical expressions include:

Addition and subtraction: Complex radical expressions can be added or subtracted if they have the same radicand. For example, $\sqrt{2} + \sqrt{2} = 2 \sqrt{2}$ .
Multiplication and division: Complex radical expressions can be multiplied or divided by multiplying or dividing their radicands. For example, $\sqrt{2} \cdot \sqrt{3} = \sqrt{6}$ and $\frac{\sqrt{8}}{\sqrt{2}} = \sqrt{4} = 2$ .
Raising to a power: A complex radical expression can be raised to a power by raising its radicand to the same power. For example, ${(\sqrt{2})}^{2} = 2$ .
Taking the nth root: The nth root of a complex radical expression can be found by taking the nth root of its radicand. For example, $\sqrt[3]{\sqrt{8}} = \sqrt[3]{8} = 2$ .

Examples of complex radical expressions include:

$\sqrt{2 + \sqrt{3}}$
$\sqrt[3]{1 + \sqrt{2}}$
$\frac{\sqrt{4 - \sqrt{5}}}{\sqrt{2}}$

Practice Problems and Solutions

Practice problems are an essential part of the learning process, as they allow students to apply their knowledge and understanding of radical functions to solve real-world problems. By working through these problems, students can identify areas where they need additional support and reinforce their understanding of the concepts.

Practice Problems

Simplify the radical expression: $\sqrt{12} \cdot \sqrt{3}$
Graph the radical function: $f (x) = \sqrt{x + 2}$
Find the inverse of the radical function: $f (x) = x^{2} - 4$
Rationalize the denominator: $\frac{1}{\sqrt{2} + 1}$

Solutions

Problem	Solution
Simplify the radical expression: $\sqrt{12} \cdot \sqrt{3}$	$\sqrt{12 \cdot 3} = \sqrt{36} = 6$
Graph the radical function: $f (x) = \sqrt{x + 2}$	The graph of $f (x) = \sqrt{x + 2}$ is a parabola that opens up and is shifted 2 units to the left.
Find the inverse of the radical function: $f (x) = x^{2} - 4$	The inverse of $f (x) = x^{2} - 4$ is $f^{- 1} (x) = \sqrt{x + 4}$ .
Rationalize the denominator: $\frac{1}{\sqrt{2} + 1}$	$\frac{1}{\sqrt{2} + 1} \cdot \frac{\sqrt{2} - 1}{\sqrt{2} - 1} = \frac{\sqrt{2} - 1}{2 - 1} = \sqrt{2} - 1$

lexy

Unit 6 Radical Functions Answer Key